A (somewhat) new paradigm for mathematics and physics | Diffusion Symmetry | N J Wildberger
Published at : November 28, 2021
The current understanding of symmetry in mathematics and physics is through group theory. However in the last 120 years, a new strand of thought has gradually appeared in a number of disciplines, from as varied as character theory, strongly regular graphs, von Neumann algebras, Hecke algebras, Lie group representation theory, cyclotomy and conformal field theory.
In this video we try to put these varied approaches together to form a unified new alternative to understanding symmetry in both mathematics and physics, taking our intuition from the diffusion of a gas in an other wise empty room -- hence the term "diffusion symmetry". We'll show through the careful analysis of a particularly rich example (familiar to all students of mathematics at some level) how both the notion of a hypergroup (going back to the 1970's with seminal papers by Dunkl, Jewett and Spector) and fusion rule algebra (appearing in physics and the study of II-1 factors and elsewhere) really do give us a rich alternative to the usual "transformational" approach to symmetry embodied in group theory.
In fact diffusion symmetry meshes very well with group theory, and sheds considerable light on many aspects of it; in particular the role of duality and character tables. By going to the diffusion point of view, we somehow miraculously replace (at least in some situations) non-commutative harmonic analysis with commutative harmonic analysis -- a major simplification.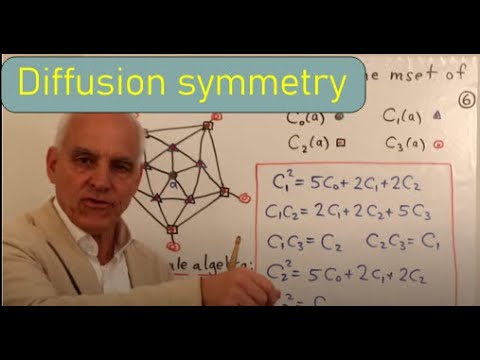
In this video we try to put these varied approaches together to form a unified new alternative to understanding symmetry in both mathematics and physics, taking our intuition from the diffusion of a gas in an other wise empty room -- hence the term "diffusion symmetry". We'll show through the careful analysis of a particularly rich example (familiar to all students of mathematics at some level) how both the notion of a hypergroup (going back to the 1970's with seminal papers by Dunkl, Jewett and Spector) and fusion rule algebra (appearing in physics and the study of II-1 factors and elsewhere) really do give us a rich alternative to the usual "transformational" approach to symmetry embodied in group theory.
In fact diffusion symmetry meshes very well with group theory, and sheds considerable light on many aspects of it; in particular the role of duality and character tables. By going to the diffusion point of view, we somehow miraculously replace (at least in some situations) non-commutative harmonic analysis with commutative harmonic analysis -- a major simplification.

(somewhat)paradigmmathematics